Slavia ZVP, Dovetail Math

I really need to make that front sight. The first thing I need to do is to measure the dovetail slot.

A caliper and a set of PeeDee thread wires.

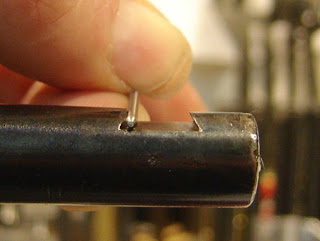
I chose a wire size that was just below the top of the slot.

With wires in either side of the dovetail I measured the width, I got .217"

But when I verified this measurement with a gage block stack it would not fit. Calipers aren't that accurate.

So I tried .216" and it was spot on.

A side view.

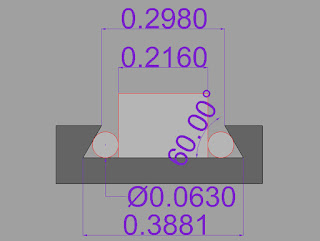
Here's a drawing.
The value we want is the width of the bottom of the slot.
We know the diameter of the pins, and the thickness of the gage block stack.
I assumed the dovetail was 60 degrees. It seemed to be.
So, knowing those and looking up the formula for measuring dovetails in Machinery's Handbook:
y=b–D(1 + cot 1⁄2α)

y=.2160
D=.063
α=60
b= base length
Since we need to know b, it becomes:
b=y+D(1 + cot 1⁄2α)

"cot" is a fancy way of saying 1/tan

tan 30 = 0.57735026918962576450914878050196
cot 30 = 1.7320508075688772935274463415059
+1=2.7320508075688772935274463415059
*D=0.17211920087683926949222911951487
+y=0.38811920087683926949222911951487

So that's how I got a value of .3881"
Honestly, I just wanted an excuse to do a little math tonight.

Short Sick Ramble

Just checking in, my creativity has been destroyed by a common cold, posting will be light this week. The good news is it's giving me plenty of time to think about how to make the sights for the ZVP...

Here are some fun links to pass the time:

• A neat article in Gun Digest about blank powered Mexican guns
• Parker and Parker Hale Scopes
• A Spring Calculator
• O-ring specialists
• Grease 101